Binomial Expansion Definition for IB SL Math AA Students
Binomial expansion is a mathematical technique used to expand binomials, which are expressions with two terms. In IB SL Math AA, binomial expansion is an important concept that is used to simplify complex mathematical expressions.
The binomial expansion formula is as follows:
(a + b)^n = C(n,0)a^n + C(n,1)a^(n-1)b + C(n,2)a^(n-2)b^2 + ... + C(n,r)a^(n-r)b^r + ... + C(n,n)b^n
where:
- (a + b) is the binomial expression that we want to expand.
- n is a positive integer representing the power to which the binomial expression is raised.
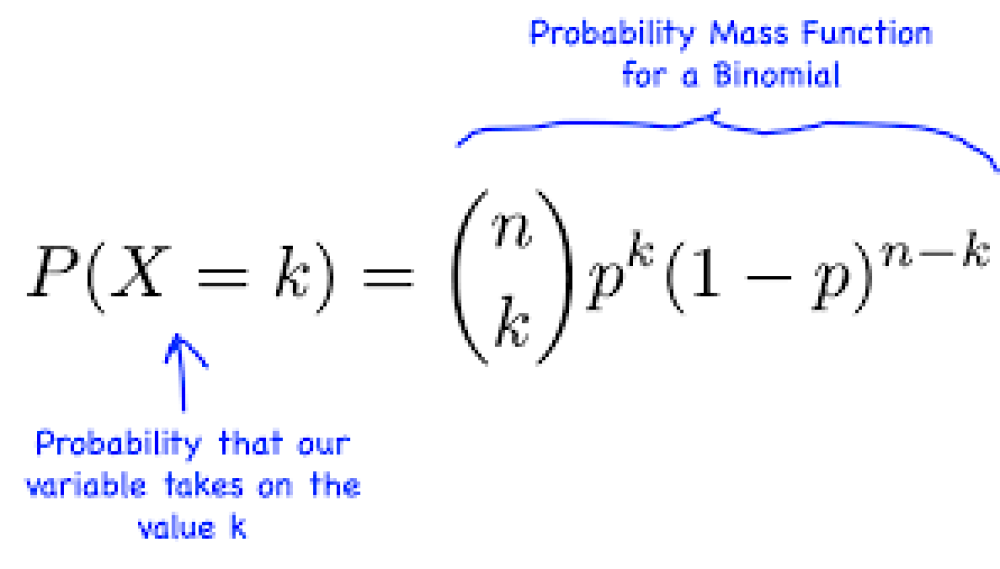
- C(n,r) is the binomial coefficient, which is the number of ways to choose r items from a set of n distinct items. It is calculated using the formula:
C(n,r) = n! / (r! * (n-r)!)
- a^n represents the term in which a appears as a factor raised to the nth power.
- a^(n-1)b represents the term in which a appears as a factor raised to the (n-1)th power and b appears as a factor raised to the 1st power.
- a^(n-2)b^2 represents the term in which a appears as a factor raised to the (n-2)th power and b appears as a factor raised to the 2nd power.
- C(n,r)a^(n-r)b^r represents the term in which a appears as a factor raised to the (n-r)th power and b appears as a factor raised to the rth power.
The formula can be interpreted as the sum of all possible ways of choosing r terms from the binomial expression (a + b) and raising each term to the appropriate power. The binomial coefficient determines the number of terms in the expansion, and each term in the expansion can be calculated using the powers of a and b and the binomial coefficient.
Binomial expansion can be used to simplify complex expressions, to find the coefficients of polynomials, and to calculate probabilities in statistics and probability theory.


