Mastering IB Math Trigonometry:
Mastering IB Math Trigonometry:
The International Baccalaureate (IB) Mathematics curriculum challenges students with a rigorous approach to various mathematical topics, and trigonometry is no exception.
Both IB Mathematics AA and AI courses have trigonometry component. And trigonometry has a key role in both curriculum.
Why Trigonometry Matters in IB Math?
Actually the heard of chapter is geometry and trigonometry. Trigonometry connects geometry and algebra. Also real-world applications used in trigonometry. From analyzing wave patterns in physics to solving real-life problems involving angles and distances, trigonometry equips you with tools to understand the world through a mathematical lens.
Core Trigonometric Concepts in IB Math
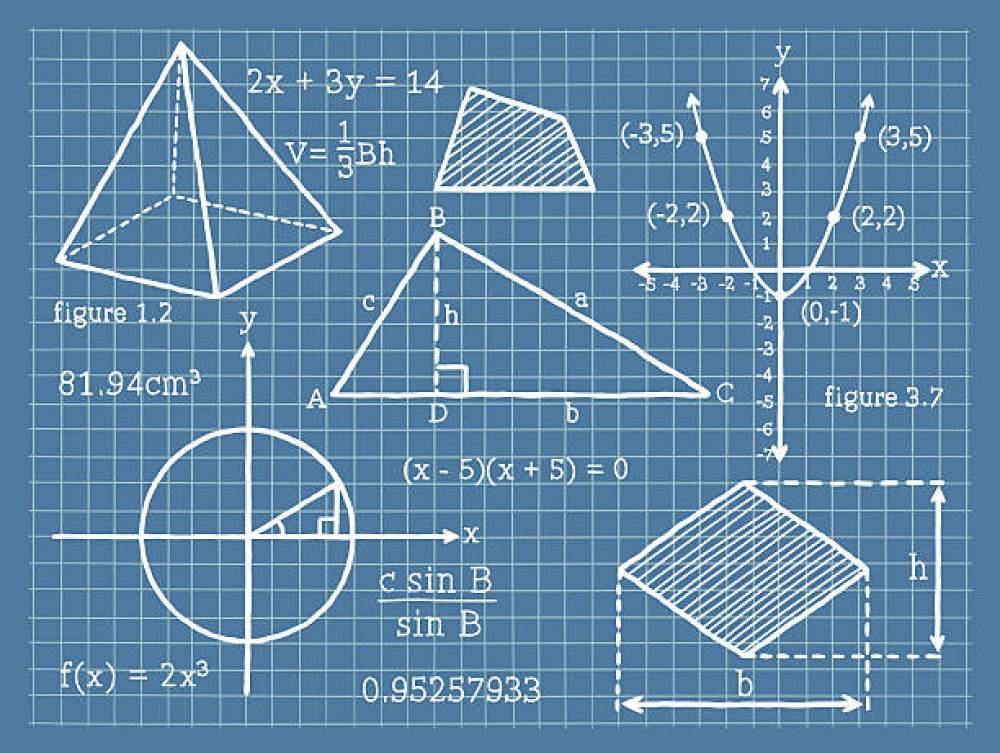
1. Unit Circle
- Essential for understanding angles beyond 90°.
- Provides a visual representation of trigonometric functions for different quadrants.
2. Trigonometric Ratios
- Sine (sin), cosine (cos), and tangent (tan) are the foundational building blocks.
- Relationships: Pythagoras' theorem leads to key identities such as .
- Reciprocal functions: Secant (sec), cosecant (csc), and cotangent (cot).
3. Trigonometric Identities
- Pythagorean identities.
- Double-angle and half-angle formulas.
- Sum and difference formulas for solving complex equations.
4. Graphing Trigonometric Functions
- Understanding periodicity, amplitude, and phase shifts.
- Graphing and identifying key transformations.
5. Solving Trigonometric Equations
- Techniques for exact solutions and numerical approximations.
- Applications in real-life scenarios, including modeling and optimization.
6. Vectors and Trigonometry
- Trigonometry is integral to vector calculations, especially when working with magnitude and direction.
Applications of Trigonometry in IB Math
- Geometry and Measurement:
- Calculating areas of triangles using .
- Finding unknown sides or angles in non-right-angled triangles using the sine and cosine rules.
- Real-Life Modeling:
- Analyzing periodic phenomena, such as tides, sound waves, and light.
- Solving problems involving circular motion and oscillations.
- Integration with Calculus:
- Trigonometric functions appear frequently in calculus topics such as differentiation and integration.
You can find common challenges and how to overcome these challenges in our next post.
Also if you want you can visit our question bank and solve questions about trigonometry.
Try our youtube page and trigonometry videos.


